Self-Consistent Calculation of Exciton Binding Energy in Quantum Structures with Rotational Symmetry
An exciton is a quasiparticle forming in semiconductors when an electron is excited from the valence band into the conduction band, leaving a positive hole behind. Due to the Coulomb interaction between opposite charge particles, the exciton has slightly less energy than the unbound electron and hole. This small difference, i.e., the binding energy, is important for precisely calculating the frequency of light emitted by the electron-hole recombination. If the quasiparticle forms inside a nanostructure, additional confinement must be taken into account. Since the exciton hamiltonian is essentially six-dimensional, calculating the binding energy is not a trivial quantum mechanics problem. Variational approximate methods do exist, but we propose here a direct FEM approach, based on a self-consistent method. In principle, our method could be applied to the most general case of asymmetric quantum dots. However, due to the prohibitive processing time involved, we restrict our analysis to quantum structures with rotational symmetry, which allows a reduction of dimensionality.
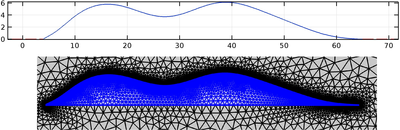
We use a pair of similar 2D axisymmetric COMSOL® models, one for the electron and the other for the hole. The models are based on the Schrödinger Equation interface in the Semiconductor Module. The Eigenvalue study was used for both particles. A real double-ring quantum structure of GaAs/AlGaAs has been chosen as physical system to be investigated (Fig. 1), for which experimental data is available as reference. Different effective masses of the particles inside and outside the ring and finite potential barriers are taken into account. Open boundary condition is imposed sufficiently far away of the ring geometry. Initially, uniparticle problems are solved independently using only the confinement potentials, for generating starting wave functions and ground levels energies for the self-consistent calculation.
The results were checked by auxiliary 3D model (Fig. 2). Then the electron model uses for each step the previous wave function of the hole, as an input for the recalculation of the equivalent confinement. Reciprocally, the hole model imports the previous electron wave function (Fig. 3), for each step. Both models exports the updated wave functions and the process continues repetitively until the energy self-consistency condition is fulfilled. The convergence within 10 μeV of the electron and hole energy sequences typically occurs after 13-17 iterations.